Cooperative Blokus
We had a fun time playing Blokus this weekend. The object of the game is to place as many pieces on the board as possible, with all your pieces touching by at least one corner and no sides. Rarely are you able to finish with all of your pieces used up, so the winner is usually the the player with the least amount of pieces left. There were four of us playing, and it ended up being a fairly close game, but it got us all wondering, what if the game were played cooperatively? Could everybody use every single piece? Could everybody win?
The board is 20 × 20 (400 squares) and each player has what amounts to 89 squares. So 89 × 4 = 356 squares. That leaves a difference of 44 squares that will not be used up. So mathematically there is enough area.
The trouble is that the pieces are all unique and unwieldy shapes so you can’t just stack them all up like the diagram. Still, it turns out the answer is yes, everybody can win.
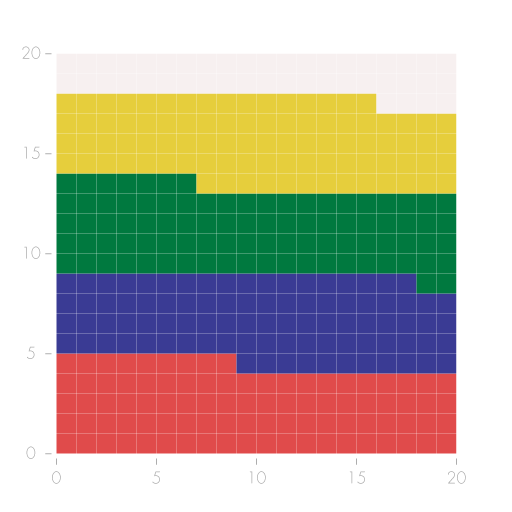
We didn’t use any mathematical formula to work it out, we simply worked off the idea that building a tessellation would be the easiest way to succeed. It was strategy mixed with intuition, trying to place pieces that allowed for as much useful area as possible in the negative spaces, and mirroring every play with each color. Here’s a photo of our finished cooperative game.
It actually doesn’t look like much of a pattern, but upon close scrutiny notice every shape that is played has it’s counterpart in every other color played in exactly the same way, but rotated ninety degrees. eg: The blue 1 × 5 piece placed in the bottom right corner has its mirrors in the other corners— green in the top right, red in the top left, and yellow on the bottom left. The symmetry is most visible in the very center of the board.
Technically this end game wouldn’t exactly be a a tessellation, but the underlying idea of symmetry is how we succeeded. Read more about tessellations on Wikipedia. Or, for fun check out the work of M.C. Escher, a master of the tessellation.